- National Curriculum Tool
- Year 5 - Multiplication and Division
Year 5 - Multiplication and Division
New Curriculum
identify multiples and factors:
- identify multiples and factors, including finding all factor pairs of a number, and common factors of 2 numbers
- know and use the vocabulary of prime numbers, prime factors and composite (non-prime) numbers
- establish whether a number up to 100 is prime and recall prime numbers up to 19
- multiply numbers up to 4 digits by a one- or two-digit number using a formal written method, including long multiplication for two-digit numbers
- multiply and divide numbers mentally, drawing upon known facts
- divide numbers up to 4 digits by a one-digit number using the formal written method of short division and interpret remainders appropriately for the context
- multiply and divide whole numbers and those involving decimals by 10, 100 and 1,000
- recognise and use square numbers and cube numbers, and the notation for squared (²) and cubed (³)
- solve problems involving multiplication and division, including using their knowledge of factors and multiples, squares and cubes
- solve problems involving addition, subtraction, multiplication and division and a combination of these, including understanding the meaning of the equals sign
- solve problems involving multiplication and division, including scaling by simple fractions and problems involving simple rates
Non-Statutory Guidance
Pupils practise and extend their use of the formal written methods of short multiplication and short division (see Mathematics Appendix 1). They apply all the multiplication tables and related division facts frequently, commit them to memory and use them confidently to make larger calculations.
They use and understand the terms factor, multiple and prime, square and cube numbers.
Pupils interpret non-integer answers to division by expressing results in different ways according to the context, including with remainders, as fractions, as decimals or by rounding (for example, 98 ÷ 4 = 98⁄4 = 24 r 2 = 24 ½ = 24.5 ≈ 25).
Pupils use multiplication and division as inverses to support the introduction of ratio in year 6, for example, by multiplying and dividing by powers of 10 in scale drawings or by multiplying and dividing by powers of a 1,000 in converting between units such as kilometres and metres.
Distributivity can be expressed as a(b + c) = ab + ac.
They understand the terms factor, multiple and prime, square and cube numbers and use them to construct equivalence statements (for example, 4 x 35 = 2 x 2 x 35; 3 x 270 = 3 x 3 x 9 x 10 = 9² x 10).
Pupils use and explain the equals sign to indicate equivalence, including in missing number problems (for example 13 + 24 = 12 + 25; 33 = 5 x ?).
Links and Resources
Pupils should make rich connections across mathematical ideas to develop fluency, mathematical reasoning and competence in solving increasingly sophisticated problems. They should also apply their mathematical knowledge to science and other subjects.
– National Curriculum, page 10
Connections within Mathematics
Making connections to other topics within this year group
Fractions (including decimals and percentages)
Requirements include:
- multiply proper fractions and mixed numbers by whole numbers, supported by materials and diagrams
- solve problems involving number up to 3 decimal places
When working on multiplication and division and/or fractions (including decimals and percentages), there are opportunities to make connections between them, for example:
You could give the children strips of paper and ask them to fold them to show you different proper and mixed fractions, for example, 5⁄8, 1 3⁄4. Next ask them to multiply these fractions by single digit numbers. They could use the strips to help them: 1 5⁄8 x 6
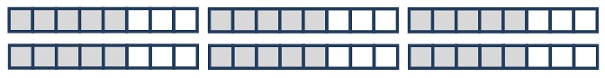
1x 6 = 6
5⁄8 x 6 = 30⁄8 or 3 6⁄8
1 5⁄8 x 6 = 6 + 3 6⁄8 = 9 6⁄8 or 9 3⁄4
Numbers with decimals are frequently seen in real life, for example when using money, so give the children opportunities to multiply these in context. For example, you could give them take-away menus and ask them to find out how much it would cost to buy four of a meal deal or a particular course. You could give them the total cost of six of the same dish and ask to work out which dish you chose.
You could ask the children problems that involve multiplying numbers up to 3 decimal places and link to measures, such as:
- Jessie had eight lengths of rope. Each was1m 36cm. If he put them side by side what would the total length be?
- Paddy had 12 cartons of orange juice. Each carton contained 0.750l. How much juice did he have altogether?
- Suzie, the baker, was making 14 loaves of bread for the local supermarket. For each loaf she needed 1.275kg of flour. What is the total amount of flour that she needed?
- India took part in a sponsored bike ride at her school. She cycled 25 times around the perimeter of the school playground. The perimeter is 105.34m. How far did she travel?
Measurement
When working on multiplication and division and/or measurement there are opportunities to make connections between them, for example:
You could give the children opportunities to rehearse multiplying by 10, 100 and1000 by converting, for example, millimetres to centimetres, centimetres to metres, metres to kilometres. They could then multiply lengths, masses and capacities of different sizes, for example, 14.75kg by 8. You could then put these into problem format, for example:
- Benji, a party organiser, was going to make a fruit punch. For each guest he needed 0.250ml of orange juice and 0.250l of mango juice. If there are 25 guests coming to the party, what is the total amount of juice Benji needs?
You could give the children an approximate equivalence between miles and kilometres, for example1.6km is approximately 1 mile. Then they multiply this amount to find approximate equivalences for other miles, for example 5 miles, 8 miles, 10 miles, 14 miles. The children could make a spider diagram for this and other equivalences.
You could give the children lengths of one side of different regular polygons, for example, pentagon, octagon, decagon, dodecagon and ask them to find their perimeters by multiplying each length by the number of sides the polygon has.
You could also give the children the lengths of different sized rectangles and ask them to find their areas, for example, a rectangle 28cm by 12cm.
Set problems involving time and money for the children to use, for example:
- Samir spent 45 minutes completing his homework. It took Pete three times as long. How long did it take Pete to complete his homework?
- It took Carol 1 ½ hours to drive from Oxford to London. It took Lorna a third of that time. How long did it take Lorna to travel to London?
- Harry is given £3.75 a week as pocket money. He is saving it to buy a computer game. How much will he have saved over 8 weeks? What about 12 weeks?
- Georgie saved £2.25 of her pocket money each week. How much will she have saved over 9 weeks?
- Penny had saved £75 over a period of 12 weeks. She saved an equal amount every week. How much did she save each week?
Making connections to this topic in adjacent year groups
Year 4
- recall multiplication and division facts for multiplication tables up to 12 × 12
- use place value, known and derived facts to multiply and divide mentally, including: multiplying by 0 and 1; dividing by 1; multiplying together 3 numbers
- recognise and use factor pairs and commutativity in mental calculations
- multiply two-digit and three-digit numbers by a one-digit number using formal written layout
- solve problems involving multiplying and adding, including using the distributive law to multiply two-digit numbers by 1 digit, integer scaling problems and harder correspondence problems such as n objects are connected to m objects
Non-Statutory Guidance
Pupils continue to practise recalling and using multiplication tables and related division facts to aid fluency.
Pupils practise mental methods and extend this to 3-digit numbers to derive facts, (for example 600 ÷ 3 = 200 can be derived from 2 x 3 = 6).
Pupils practise to become fluent in the formal written method of short multiplication and short division with exact answers (see Mathematics Appendix 1).
Pupils write statements about the equality of expressions (for example, use the distributive law 39 × 7 = 30 × 7 + 9 × 7 and associative law (2 × 3) × 4 = 2 × (3 × 4)). They combine their knowledge of number facts and rules of arithmetic to solve mental and written calculations for example, 2 x 6 x 5 = 10 x 6 = 60.
Pupils solve two-step problems in contexts, choosing the appropriate operation, working with increasingly harder numbers. This should include correspondence questions such as the numbers of choices of a meal on a menu, or 3 cakes shared equally between 10 children.
Year 6
- multiply multi-digit numbers up to 4 digits by a two-digit whole number using the formal written method of long multiplication
- divide numbers up to 4 digits by a two-digit whole number using the formal written method of long division, and interpret remainders as whole number remainders, fractions, or by rounding, as appropriate for the context
- divide numbers up to 4 digits by a two-digit number using the formal written method of short division where appropriate, interpreting remainders according to the context
- perform mental calculations, including with mixed operations and large numbers
- identify common factors, common multiples and prime numbers
- use their knowledge of the order of operations to carry out calculations involving the 4 operations
- solve addition and subtraction multi-step problems in contexts, deciding which operations and methods to use and why
- solve problems involving addition, subtraction, multiplication and division
- use estimation to check answers to calculations and determine, in the context of a problem, an appropriate degree of accuracy
Non-Statutory Guidance
Pupils use the whole number system, including saying, reading and writing numbers accurately Pupils practise addition, subtraction, multiplication and division for larger numbers, using the formal written methods of columnar addition and subtraction, short and long multiplication, and short and long division (see Mathematics Appendix 1).
They undertake mental calculations with increasingly large numbers and more complex calculations.
Pupils continue to use all the multiplication tables to calculate mathematical statements in order to maintain their fluency.
Pupils round answers to a specified degree of accuracy, for example, to the nearest 10, 20, 50, etc, but not to a specified number of significant figures.
Pupils explore the order of operations using brackets; for example, 2 + 1 x 3 = 5 and (2 + 1) x 3 = 9.
Common factors can be related to finding equivalent fractions.
Cross-curricular and real life connections
Learners will encounter number and place value in:
Within the geography curriculum there are opportunities to connect with multiplication and division, for example in the introduction of the Key Stage 2 Programme of Study it states that pupils should extend their knowledge and understanding beyond the local area to include the United Kingdom and Europe, North and South America. This will include the location and characteristics of a range of the world’s most significant human and physical features. Children could, for example, find out about the currencies used in a selection of countries. They could then make up a currency converter using mental calculation strategies and then check using multiplication, for example:
£1= 1.20 Euros
£2 = 2.40 Euros
£3 = 3.60 Euros
£4 = 4.80 Euros
£5 = 6 Euros
| Programme of Study statements | Activities | |||||||
|---|---|---|---|---|---|---|---|---|
| A | B | C | D | E | F | G | H | |
| identify multiples and factors, including finding all factor pairs of a number, and common factors of 2 numbers | ||||||||
| know and use the vocabulary of prime numbers, prime factors and composite (non-prime) numbers | ||||||||
| establish whether a number up to 100 is prime and recall prime numbers up to 19 | ||||||||
| multiply numbers up to 4 digits by a one- or two-digit number using a formal written method, including long multiplication for two-digit numbers | ||||||||
| multiply and divide numbers mentally, drawing upon known facts | ||||||||
| divide numbers up to 4 digits by a one-digit number using the formal written method of short division and interpret remainders appropriately for the context | ||||||||
| multiply and divide whole numbers and those involving decimals by 10, 100 and 1,000 | ||||||||
| recognise and use square numbers and cube numbers, and the notation for squared (²) and cubed (³) | ||||||||
| solve problems involving multiplication and division, including using their knowledge of factors and multiples, squares and cubes | ||||||||
| solve problems involving addition, subtraction, multiplication and division and a combination of these, including understanding the meaning of the equals sign | ||||||||
| solve problems involving multiplication and division, including scaling by simple fractions and problems involving simple rates | ||||||||
Activity set A
You could write a number on the board, such as, 6 and ask the children to write down as many multiples of six as they can in one or two minutes. Encourage them to think of multiples that come in the multiplication table for 6 and others, for example, 720, 360, 1440. Once they have done this ask them to look at what they wrote and to identify other numbers that these are multiples of so finding common multiples.
You could do something similar for factors. Write a number, such as 144 on the board and give the children two minutes to find all the factors they can of 144. Once they have done this, ask them to look at the factors and to find another number that each is a factor of.
Take a look at the Nrich problem ‘Factors and multiples puzzle’. It is quite challenging as it stands but can be adapted to suit most attainment levels, for example, you might like to provide a list of numbers for children to put into the correct cells in the table:
| Multiple of 3 | Factor of 24 | Multiple of 5 | Factor of 72 | |
| Even Number | ||||
| Odd Number | ||||
| Multiple of 8 | ||||
| Factor of 120 |
You could then ask the children to think of other numbers to add to each section.
Activity set B
Remind the children that a prime number is a number that can only be divided by one and itself. So a prime number has two factors. You could give the children a 100 square and ask them to shade the numbers from 1to10 that are not prime numbers (1, 4, 6, 8, 10). They then shade all the multiples of 2, 3, 4, 5, 6, 7, 8, 9 and 10. The numbers that are left unshaded are all primes. You could ask them what they notice about these (they are mostly either side of multiples of six).
You could write some single and 2-digit numbers on the board and ask the children to break these down as much as they can. What do they notice? If they have broken them down as far as possible they will end up with prime factors. Here is an example: 24 – factors include 2 and 12, 2 is a prime factor. Factors of 12 include 3 and 4, 3 is a prime. Factors of 4 include 2 and 2, both of which are prime factors. So, the prime factors of 24 are 2, 2, 2 and 3.
You could carry out similar activities for composite numbers.
Activity set C
You could display a variety of multiplication and division calculations on the board and ask the children to decide which strategy they would use to answer them. They could then discuss their thinking with a partner. Encourage them to look at the numbers and decide whether they can use a mental calculation strategy, jottings or a written method. Here are some examples of questions you could use and some possible appropriate strategies:
- 24 x 50 (x 100 and halve)
- 52 x 4 (double and double again)
- 12 x 15 (x 10, halve and total x10 and half x10)
- 136 x 9 (partitioning, x10 and take away 136 or column method)
- 245 x 1.6 (grid method or the column method or x1, x half, x tenth and add together)
- 123 x 3 (re-partition number into 120 and 3, 4 x 3 = 12 so 40 x 3 = 120 (so 120 ÷ 3 = 40), 3 ÷ 3 = 1, answer 41)
- 165 x 10 (make number ten times smaller)
- 325 x 25 (use knowledge that there are four 25s in 100)
- 408 x 17 (grouping in 17s, 20 groups make 340, 4 groups make 68 so answer is 24)
- 623 x 9 (short method)
You could try ‘All the digits’ The multiplication given uses each of the digits 0 - 9 once and once only. Using the information given, the children need to replace the stars in the calculation with figures.
You could give the children a set of calculations which have been answered using column method and ask them to look at them and decide which are easy and which are difficult and why.
Activity set D
Give the children place value grids similar to the one below and a set of digit cards with some extra zeros:
| 1000 | 100 | 10 | 1 | . | 10th | 100th |
Ask them to make a three digit number, such as 34.8, and place it in the grid. They can then multiply the number by 10 and 100 using zeros as place holders and describe what is happening: the number is becoming 10/100 times bigger, the digits are moving to the left.
They could then divide their number by 10, 100 and 1000 and describe what is happening: the number is becoming 10/100/1000 times smaller, the digits are moving to the right.
Activity set E
You could give the children centimetre squared paper and ask them to explore square numbers by drawing squares 1 x 1, 2 x 2, 3 x 3 etc. Ask them what they notice. Encourage them to notice that a square is made with sides of equal lengths and that to find the area they multiply the length by the width so giving 12, 22, 32 and so on. These are known as square numbers. Can they work out the formula for the area of a square: n2. Give them a variety of numbers to represent ‘n’.
Ask the children to list as many square numbers as they can in ascending order in two minutes.
Give the children a centimetre cube. Ask them to work out the volume by multiplying the length, width and height. Next, ask them to build another cube with three dimensions of 2cm. They work out the volume of this and then explore other cubes. What do they notice? Encourage them to notice that each cube has three dimensions of the same size. When multiplied they produce cubed numbers: 13 is 1 x 1 x 1 = 1, 23 is 2 x 2 x 2 = 8, 33 is 3 x 3 x 3 = 27 and so on.
Ask the children to list as many cubed numbers as they can in ascending order in two minutes.
Stand a container (tank or bowl or bucket) inside another container ( a larger bowl or a tray with sides at least a few centimeters high). Fill the container to the brim with water. Place the 1000 Dienes cube (or equivalent) into the container. Catch and measure the volume of water that overflows (is displaced). What do you notice?
Activity set F
You could ask the children to solve problems such as:
- Sally was asked to find all the factors of 48. She found 8. These were, 1, 48, 2, 24, 3, 16, 4, 12. Did she find them all? How do you know?
- Bobby was asked to find all the multiples of 12. He said that it was impossible because there were an infinite number. Was he correct? Explain your thinking.
- Farmer Giles bought a plot of land. It was a square shape with a perimeter of 48m. What was its area? He paid £56 for each square metre. How much did he pay in total?
- Fatima bought a microwave for her kitchen. It was cube shaped and had a length of 30cm. How much space did it take up?
Activity set G
Give the children algebraic type problems that involve balancing to help them understand the meaning of the equals sign. For example:
- 2n + 10 = 36 (take 10 from each side to give 2n = 26, divide each side by 2 to give n = 13
- 7 = 2x ÷ 6 (multiply each side by 6 to give 7 x 6 = 2x which is 42 = 2x, divide each side by 2 to give 21 = x
You could also give problems such as:
- Sharon and Tim each had a collection of football stickers. Tim had 5 times as many as Sharon. He had 150. How many did they have altogether?
You could encourage the children to use the bar model to solve this:
Sharon
Tim
Tim has 150 stickers, so each square represents 30 stickers. Therefore Sharon has 30 and altogether they have 180.
- Tina had a cupboard in her bedroom on which she kept her books. There were 15 books on each of 8 shelves. A friend gave her another 24 books which she put equally onto the 8 shelves. How many books were on each shelf?
Activity set H
You could show photographs of some famous buildings or the children to illustrate how objects or people are scaled down. Explain that, to describe how much something has been scaled down, we often use ratio or simple fractions.
You could set this problem: A tennis court is 7m wide and 24m long. A scale plan of it is drawn with a width of 3.5cm. What is its length? Agree that 7m has been divided by 100 to become centimetres and then halved. The same must therefore be done with 24m to give 12cm. You could repeat this type of problem with other similar scenarios.
The children could work in a small group to make 2D drawings of objects in the classroom. They measure heights and widths of their objects and then scale them down. They decide their own ratio for scaling down, for example, 1:2 (half the size) or 1:3 (one third of the size). Make the point that scaling down is the same as multiplying by a value less than 1.
Examples of what children should be able to do, in relation to each (boxed) Programme of Study statement
identify multiples and factors, including finding all factor pairs of a number, and common factors of 2 numbers
know and use the vocabulary of prime numbers, prime factors and composite (non-prime) numbers
establish whether a number up to 100 is prime and recall prime numbers up to 19
- Use the vocabulary factor, multiple and product. They identify all the factors of a given number; for example, the factors of 20 are 1, 2, 4, 5, 10 and 20. They answer questions such as:
- Find some numbers that have a factor of 4 and a factor of 5. What do you notice?
- My age is a multiple of 8. Next year my age will be a multiple of 7. How old am I?
- They recognise that numbers with only two factors are prime numbers and can apply their knowledge of multiples and tests of divisibility to identify the prime numbers less than 100. They explain that 73 children can only be organised as 1 group of 73 or 73 groups of 1, whereas 44 children could be organised as 1 group of 44, 2 groups of 22, 4 groups of 11, 11 groups of 4, 22 groups of 2 or 44 groups of 1. They explore the pattern of primes on a 100-square, explaining why there will never be a prime number in the tenth column and the fourth column.
multiply and divide numbers mentally, drawing upon known facts
- Rehearse multiplication facts and use these to derive division facts, to find factors of two-digit numbers and to multiply multiples of 10 and 100, e.g. 40 × 50. They use and discuss mental strategies for special cases of harder types of calculations, for example to work out 274 + 96,< 8006 – 2993, 35 × 11, 72 ÷ 3, 50 × 900. They use factors to work out a calculation such as 16 × 6 by thinking of it as 16 × 2 × 3. They record their methods using diagrams (such as number lines) or jottings and explain their methods to each other. They compare alternative methods for the same calculation and discuss any merits and disadvantages.
multiply numbers up to 4 digits by a one- or two-digit number using a formal written method, including long multiplication for two-digit numbers
- Develop and refine written methods for multiplication. They move from expanded layouts (such as the grid method) towards a compact layout for HTU × U and TU × TU calculations. They suggest what they expect the approximate answer to be before starting a calculation and use this to check that their answer sounds sensible. For example, 56 × 27 is approximately 60 × 30 = 1800.
multiply and divide whole numbers and those involving decimals by 10, 100 and 1,000
- Recall quickly multiplication facts up to 10 × 10 and use them to multiply pairs of multiples of 10 and 100. They should be able to answer problems such as:
- the product is 400. At least one of the numbers is a multiple of 10. What two numbers could have been multiplied together? Are there any other possibilities?
recognise and use square numbers and cube numbers, and the notation for squared (²) and cubed (³)
- solve problems involving multiplication and division, including using their knowledge of factors and multiples, squares and cubes
- use knowledge of multiplication facts to derive quickly squares of numbers to 12 × 12 and the corresponding squares of multiples of 10. They should be able to answer problems such as:
- tell me how to work out the area of a piece of cardboard with dimensions 30 cm by 30 cm
- find two square numbers that total 45
divide numbers up to 4 digits by a one-digit number using the formal written method of short division and interpret remainders appropriately for the context
- Extend written methods for division to include HTU ÷ U, including calculations with remainders. They suggest what they expect the approximate answer to be before starting a calculation and use this to check that their answer sounds sensible. They increase the efficiency of the methods that they are using. For example:
196 ÷ 6 is approximately 200 ÷ 5 = 40
3 2 r4 or 4/6 or 2/3
6 196
Children know that, depending on the context, answers to division questions may need to be rounded up or rounded down. They explain how they decided whether to round up or down to answer problems such as:
- Egg boxes hold 6 eggs. A farmer collects 439 eggs. How many boxes can he fill
- Egg boxes hold 6 eggs. How many boxes must a restaurant buy to have 200 eggs?
solve problems involving addition, subtraction, multiplication and division and a combination of these, including understanding the meaning of the equals sign
solve problems involving multiplication and division, including scaling by simple fractions and problems involving simple rates
- Use written methods to solve problems and puzzles such as:
275 382 81 174 206 117 414 262 483 173 239 138 331 230 325 170 - Choose any four numbers from the grid and add them. Find as many ways as possible of making 1000.
- Place the digits 0 to 9 to make this calculation correct: ☐☐☐☐ – ☐☐☐ = ☐☐☐.
- Two numbers have a total of 1000 and a difference of 246. What are the two numbers?