National Curriculum Resource Tool

;

National Curriculum Resource Tool

Materials to support teachers and schools in embedding the National Curriculum

This page, and all sections of the National Curriculum Resource Tool, will be removed in summer 2024. Find out more

National Curriculum Tool
Year 5 - Number and Place Value

Year 5 - Number and Place Value

New Curriculum

read, write, order and compare numbers to at least 1 000 000 and determine the value of each digit
count forwards or backwards in steps of powers of 10 for any given number up to 1 000 000
interpret negative numbers in context, count forwards and backwards with positive and negative whole numbers, including through zero
round any number up to 1 000 000 to the nearest 10, 100, 1000, 10 000 and 100 000
solve number problems and practical problems that involve all of the above
read Roman numerals to 1000 (M) and recognise years written in Roman numerals.

Non-Statutory Guidance

Pupils identify the place value in large whole numbers.

They continue to use number in context including measurement. Pupils extend and apply their understanding of the number system to the decimal numbers and fractions that they have met so far.

They should recognise and describe linear number sequences (for example 3, 3½, 4, 4½ …) including those involving fractions and decimals and find the term-to-term rule in words (for example add ½).

Links and Resources

Pupils should make rich connections across mathematical ideas to develop fluency mathematical reasoning and competence in solving increasingly sophisticated problems. They should also apply their mathematical knowledge to science and other subjects.

– National Curriculum, page 10

Connections within Mathematics

Making connections to other topics within this year group

Addition and subtraction

add and subtract whole numbers with more than 4 digits including using formal written methods (columnar addition and subtraction)
add and subtract numbers mentally with increasingly large numbers
use rounding to check answers to calculations and determine in the context of a problem levels of accuracy

When working on number and place value and/or addition and subtraction there are opportunities to make connections between them for example:

Numbers with decimals are frequently seen in real life, so give the children opportunities to add and subtract these in context. For example, you could give them catalogues or take away menus and ask them to choose two or three items to buy. You could give them a budget and ask them total the prices and find out how much of their budget is left.

You could ask the children to measure the lengths of different objects around the classroom and to find their total length. They could then represent these measurements in centimetres and metres. They could then convert them into metre measurements using decimals, for example 3m 24cm would become 3.24m. You could ask them to find out what length they would need to make a longer length that you give them, such as 10m. They could do similar activities for volume and capacity and also mass.

Encourage the children to consider whether a mental calculation strategy or a written strategy would be most efficient for their additions and subtractions. They could also make estimates of the totals and differences using rounding.

Multiplication and division

multiply numbers up to 4 digits by a one- or two-digit number using a formal written method including long multiplication for two-digit numbers
multiply and divide numbers mentally drawing upon known facts
divide numbers up to 4 digits by a one-digit number using the formal written method of short division and interpret remainders appropriately for the context
multiply and divide whole numbers and those involving decimals by 10 100 and 1 000

When working on number and place value and/or multiplication and division there are opportunities to make connections between them, for example:

You could make up problems for the children to solve that involve multiplication and division for example:

Harris had £38. 96. He shared his money into four equal piles. How much money was in each pile?
Naomi was making some fruit juice for a party. She decided each person would need 350ml of juice. If there were 24 people at the party, how many litres of juice does she need to make?

Give the children place value grids similar to the one below and a set of digit cards:

1000	100	10	1	.	1/10	1/100

Ask them to make a three digit number, such as 569 and place it in the grid. They can then multiply the number by ten, using the zero as a place holder. They could then divide their number by 10, 100 and 1000 and describe what is happening: the number is becoming 10/100/1000 times smaller the digits are moving to the right.

Fractions (including decimals and percentages)

recognise and use thousandths and relate them to tenths hundredths and decimal equivalents
round decimals with two decimal places to the nearest whole number and to one decimal place
read write order and compare numbers with up to three decimal places
solve problems involving number up to three decimal places

When working on number and place value and/or fractions (including decimals and percentages) there are opportunities to make connections between them for example:

Using the place value grid suggested above and digit cards, ask the children to make a number that fills the grid. Discuss what each digit is worth. For example, with the number 2315.67 the 2 is in the thousands position so that tells us how many thousands it represents – so the value shown in that column is 2000 the 3 is in the hundreds position so is 300 and so on. When you discuss the 6 and 7 ensure that the children recognise that the 6 is in the tenths position so is worth 6/10 or 0.6 and the 7 is in the hundredths position so is worth 7/100 or 0.07. They could write the numbers they make in words so that they reinforce their place value. They should also model them with structured base 10 apparatus.

You could take five examples of the numbers that the children have made in their grids write them on the board and then ask the children to order them in ascending or descending order.

Ask problems involving mass, for example:

Charlie has three cats. Macy weighs 3kg 250g , Tia weighs 2kg 175g and Elvis weighs 4kg 125g. What would these masses be in kilograms only? In kilograms work out the total mass of the three cats?
Georgie was making a cake; she needed 1.6kg of flour 350g of butter and 750g of sugar. What is the total mass of these ingredients?
Samir made five jugs of juice. For each he used 2 litres of water and 245ml of cordial. How many litres of liquid did he use altogether.

Measurement

convert between different units of metric measure [for example kilometre and metre; centimetre and metre; centimetre and millimetre; gram and kilogram; litre and millilitre]

When working on number and place value and/or measures there are opportunities to make connections between them, for example:

Give the children a list of different metric units and ask them to write them in different ways. For example:

3km 50m could also be written as 3050m or 3.05km
2m 10cm could also be written as 210cm or 2.1m
13cm 7mm could also be written as 137mm or 13.7cm
6l 75ml could also be written as 6075ml or 6.075l

Using maps the children could work out distances to different places. These are likely to have a scale in centimetres. The children could convert these to kilometres to find the actual distances.

Making connections to this topic in adjacent year groups

Year 4

count in multiples of 6 7 9 25 and 1 000
find 1 000 more or less than a given number
count backwards through zero to include negative numbers
recognise the place value of each digit in a four-digit number (thousands hundreds tens and ones)
order and compare numbers beyond 1 000
identify represent and estimate numbers using different representations
round any number to the nearest 10 100 or 1 000
solve number and practical problems that involve all of the above and with increasingly large positive numbers
read Roman numerals to 100 (I to C) and know that over time the numeral system changed to include the concept of zero and place value

Non-statutory guidance

Using a variety of representations including measures pupils become fluent in the order and place value of numbers beyond 1 000 including counting in 10s and 100s and maintaining fluency in other multiples through varied and frequent.

They begin to extend their knowledge of the number system to include the decimal numbers and fractions that they have met so far.

They connect estimation and rounding numbers to the use of measuring instruments.

Roman numerals should be put in their historical context so pupils understand that there have been different ways to write whole numbers and that the important concepts of zero and place value were introduced over a period of time.

Year 6

read write order and compare numbers up to 10 000 000 and determine the value of each digit
round any whole number to a required degree of accuracy
use negative numbers in context and calculate intervals across zero
solve number and practical problems that involve all of the above

Non-statutory guidance

Pupils use the whole number system including saying reading and writing numbers accurately.

Cross-curricular and real life connections

Within the science curriculum there are opportunities to work with number and place value, for example, in the introduction of the Upper Key Stage 2 Programme of Study it states that pupils should select the most appropriate ways to answer science questions using different types of scientific enquiry including observing changes over different periods of time noticing patterns grouping and classifying things carrying out comparative and fair tests and finding things out using a wide range of secondary sources of information. The children could, for example, record changes over periods of time and compare them. You could discuss the differences in the place value of periods of time and the number system. They could record, for example, heights of plants accurately using decimal notation.

Within the geography curriculum there are opportunities to connect with number and place value for example in the introduction of the Key Stage 2 Programme of Study it states that pupils should extend their knowledge and understanding beyond the local area to include the United Kingdom and Europe North and South America. This will include the location and characteristics of a range of the world’s most significant human and physical features. Children could, for example, find and compare distances between countries or cities temperatures lengths of rivers heights of mountains. These comparisons will involve finding differences which involve a secure understanding of place value.

See, for example:

Within the history curriculum there are opportunities to work with number and place value for example in the introduction of the Key Stage 2 Programme of Study it states that pupils should continue to develop a chronologically secure knowledge and understanding of British local and world history establishing clear narratives within and across the periods they study. The children could, when studying the Roman period, focus on their number system and find out how it developed. A Little bit of History in issue 2 of the Primary Magazine has information about this. They could also look at the development of our number system. A Little bit of History in issue 8 of the Primary Magazine has information about this.

“Putting place value in its place” is a thought-provoking article by Ian Thompson.
Another is “Teaching Place Value in the UK: time for a reappraisal?” published in Education Review in 2000.
Using calculators for assessing pupils’ conceptualization on place-value Papadopoulos Ioannis 2013. This paper is a two-stage research study focused on problem solving relevant to place value and on the use of the operations within the calculator environment. The findings show the use of calculators and appropriate tasks within a context help Year 5 or 6 pupils to apply their understanding of place value. Note: this article is behind a paywall on an external site, not 'free-to-view' as stated.

Programme of Study statements	Activities
Programme of Study statements	A	B	C	D	E	F
read, write, order and compare numbers to at least 1 000 000 and determine the value of each digit
count forwards or backwards in steps of powers of 10 for any given number up to 1 000 000
interpret negative numbers in context count forwards and backwards with positive and negative whole numbers including through
round any number up to 1 000 000 to the nearest 10, 100, 1000, 10 000 and 100 000
solve number problems and practical problems that involve all of the above
read Roman numerals to 1000 (M) and recognise years written in Roman numerals

Activity set A

It is important that the children understand the place value of different digits. Conceptually, place value is complex and difficult for children to learn. Sometimes we assume children understand this concept if they can partition, say, 1345 into 1000 + 300 + 40 + 5. This isn’t necessarily so. Place value needs to be understood in four important ways: ‘positional’ ‘multiplicative’ additive’ and ‘base 10’.

Display a grid similar to this on the board:

1 000 000	100 000	10 000	1000	100	10	1	.	¹⁄₁₀	¹⁄₁₀₀	¹⁄₁₀₀₀
6	8	2	4	2	5	7	.	9	3	5

Ask the children to explain what each digit is. For example the 2 is in the 10 000 column (positional) to find the number it represents we multiply it by 10 000 to give 20 000 (multiplicative). The 7 is in the ones column (positional); to find what the number 7 represents we multiply by one to give 7 (multiplicative). The 3 is in the hundredths column (positional) to find the number it represents we multiply it by one hundredth to give 3/100 (multiplicative). When we put the digits together to give the total value we must add the values represented in each of the columns together to know the total value represented 6 824 157 . 935 (additive). Each digit represents a number that is either 10 times larger or 10 times smaller than the values in adjacent columns (base 10).

You could give the children a set of digit cards and ask them to make and read large numbers following instructions that you call out such as these: make 34 now 234 now 2348, 23 487, 123 487, 9 123 487. Show the cards that show how many hundreds, tens, millions, thousands etc. there are.

They could then swap different digits and say whether the number is now bigger or smaller and by roughly how much- for example, 9 123 487 swap the 2 and 8: the number is bigger by roughly 60 thousand.

They could select four, five or six digit cards and make the highest and lowest number and the one closest to 5000.

The children could do a similar activity on their whiteboards. This has the added bonus of writing the numbers as well.

You could give the children problems such as:

Freddy scored 28 456 points on the computer game. His friend Hugh scored 5000 points more than Freddy. How many points did Hugh score?
There were 85 356 people at the Liverpool match. There were 40 000 fewer people at the Manchester United match. How many people were at the Man U match?
A London post office delivered 1 750 000 Christmas cards on the Monday before Christmas and 300 000 more on the Tuesday. How many did the post office deliver on the Tuesday?

You could explore place value with a calculator. Ask the children to key in a six digit number for example 234 568. Next give them instructions such as ‘change the 4 into a 9’, ‘change the 2 into a 7’. Each time ask them to explain what they did (take 4000 and add 9000 or add 5000 take 200 000 away and add 700 000 or add 500 000).

Activity set B

The children could write numbers on their whiteboards and then make them 10, 100, 1000 times larger.

You could use a pendulum (easily made from three multilink cubes and a (long) piece of string) to mark time while children practise counting on or back in steps of different 10, 100, 1000 and 1million from and to a given number e.g. from 75 to 175/1075/10 075 etc. You could also do this for tenths, hundredths and thousandths.

You could use a counting stick and count forwards and backwards in steps of thousandths, hundredths, tenths 10 100 1000 etc. You could start by telling them that zero is at one end and for example 10 000 at the other. The children then need to work out what equal steps they need to count in to get from one end to the other. Be sure to jump around the counting stick to keep the children on their toes!

You could ask questions as if the counting stick was a number line; for example, what would go on this division what about half way between each end?

You could give the children this Nrich activity The Thousands Game

Activity set C

You could show the ITP (Interactive Teaching Programme from National Strategies) Thermometer. Set the maximum temperature at 500, the minimum at -300 and the interval at 2. You could then ask volunteers to show different temperatures. The children could work out differences between: two negative temperatures; a negative and a positive temperature; and two positive temperatures.

Discuss in which countries or regions of the world negative temperatures are found and how cold it can get in these places. You can find some information on this in a feature on Polar Regions in the Primary Magazine.

Activity D

Ask the children to draw different number lines that would enable them to identify a number that would be rounded to 10 100 1000 10 000 or 100 000. For example:

Round 1346 to the nearest 10

1346 is closest to 1350.

Round 1346 to the nearest 100

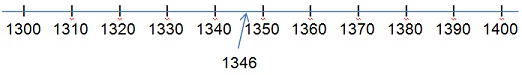
1346 is closest to 1300.

Round 1346 to the nearest 1000

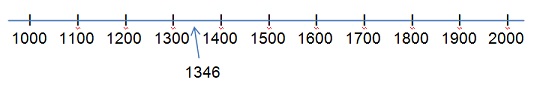
1346 is closest to 1000.

Activity set E

You could ask the children problems which involve approximate answers that can be found by rounding, for example:

Becky wanted to buy some clothes. The jeans she wanted cost £48.75, the sweat shirt cost £29.99, the trainers cost £59.80. She has saved up £150. Does she have enough money to buy the clothes?
Sam was taking a survey of the number of cars being driven down the High Street over a four hour period. These were his results: 1st hour 219 cars 2nd hour 498 cars 3rd hour 314 cars 4th hour 189 cars. To the nearest hundred how many cars did he record?

You could ask the children problems involving positive and negative numbers for example:

The temperature in Reykjavik at 6am was -12°C. During the day the temperature rose by 18 degrees. What was the new, higher temperature?
The average annual temperature in the Antarctica is -57°C. The average annual temperature in the Maldives is 27°C. What is the difference between these two averages?

Activity set F

You could give the children a table showing the basic Roman numerals follow a pattern:

Units	I	II	III	IV	V	VI	VII	VIII	IX
Tens	X	XX	XXX	XL	L	LX	LXX	LXXX	XC
Hundreds	C	CC	CCC	CD	D	DC	DCC	DCCC	CM
Thousands	M	MM	MMM	IV	V	VII	VII	VIII	IX

Ask the children to use the table to make up different 4 digit Roman numbers for example 2365 or the year they were born or the year we are in now.

You could write some of these on the board and ask the children to convert them to ‘our’ numbers for example MCDLXIV.

A Little Bit of History in issue 2 of the Primary Magazine gives details of how to write Roman Numerals.

Examples of what children should be able to do, in relation to each (boxed) Programme of Study statement

read, write, order and compare numbers to at least 1 000 000 and determine the value of each digit

Explain what each digit represents in whole numbers and decimals with up to two places and partition, round and order these numbers.
Answer problems such as
- What is the value of the 7 in 3 274 105?
- Write in figures forty thousand and twenty.
- A number is partitioned like this:
  4 000 000 + 200 000 + 60 000 + 300 + 50 + 8
  
  Write the number. Now read it to me.
- A car costs more than £8600 but less than £9100. Tick the prices that the car might cost.
  £8569 □ £9090 □ £9130 □ £8999 □

count forwards or backwards in steps of powers of 10 for any given number up to 1 000 000

Count from any given number in powers of 10 and decimal steps extending beyond zero when counting backwards; relate the numbers to their position on a number line
Answer problems such as:
- Write the next number in this counting sequence: 110 000, 120 000, 130 000 …
- Create a sequence that goes backwards and forwards in tens and includes the number 190. Describe your sequence.
- Here is part of a sequence: 30, 70, 110, □, 190, □. How can you find the missing numbers?

interpret negative numbers in context, count forwards and backwards with positive and negative whole numbers, including through 0

Count from any given number in whole-number and decimal steps extending beyond zero when counting backwards; relate the numbers to their position on a number line.

round any number up to 1 000 000 to the nearest 10 100 1 000 10 000 and 100 000

Explain what each digit represents in whole numbers and decimals with up to two places and partition round and order these numbers and answer questions such as: What is 4773 rounded to the nearest hundred?

solve number problems and practical problems that involve all of the above

Partition decimals using both decimal and fraction notation for example recording 6.38 as 6 + ³⁄₁₀ + ⁸⁄₁₀₀ and as 6 + 0.3 + 0.08. They write a decimal given its parts: e.g. they record the number that is made from 4 wholes 2 tenths and 7 hundredths as 4.27. They apply their understanding in activities such as:
- Find the missing number in 17.82 – □ = 17.22.
- Play ‘Zap the digit’: In pairs choose a decimal to enter into a calculator e.g. 47.25. Take turns to ‘zap’ (remove) a particular digit using subtraction. For example to ‘zap’ the 2 in 47.25 subtract 0.2 to leave 47.05.
The children explain how they work out calculations showing understanding of the place value that underpins written methods.

read Roman numerals to 1000 (M) and recognise years written in Roman numerals

Recognise Roman numerals in their historical context
Read and write Roman numerals to one thousand

The Decimal Place 1

The video ‘The Decimal Place 1’ shows Pamela Morgan helping her Y4/5 class extend their understanding of decimals.

The Decimal Place 2

The video ‘The Decimal Place 2’ is a discussion about the lesson featured in ‘The Decimal Place 1’.

Is there anything wrong with this page?

Subscribe to our newsletter

The NCETM is led and delivered by Tribal Education Services, with MEI as a key partner. Learn more about Tribal Education Services and what they do via the link to their website in 'About the NCETM'.