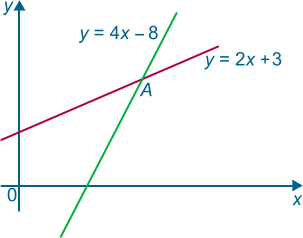This page, and all sections of the National Curriculum Resource Tool, will be removed in summer 2024. Find out more
- National Curriculum Tool
- KS3 - Algebra
KS3 - Algebra
New Curriculum
Pupils should be taught to:
- Use and interpret algebraic notation, including:
- ab in place of a × b
- 3y in place of y + y + y and 3 × y
- a2 in place of a × a, a3 in place of a × a × a; a2b in place of a × a × b
- ab in place of a ÷ b
- coefficients written as fractions rather than as decimals
- brackets
- substitute numerical values into formulae and expressions, including scientific formulae
- understand and use the concepts and vocabulary of expressions, equations, inequalities, terms and factors
- simplify and manipulate algebraic expressions to maintain equivalence by:
- collecting like terms
- multiplying a single term over a bracket
- taking out common factors
- expanding products of two or more binominals
- understand and use standard mathematical formulae; rearrange formulae to change the subject
- model situations or procedures by translating them into algebraic expressions or formulae and by using graphs
- use algebraic methods to solve linear equations in one variable (including all forms that require rearrangement)
- work with coordinates in all four quadrants
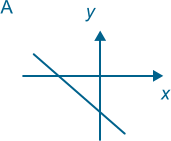
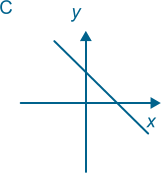
- recognise, sketch and produce graphs of linear and quadratic functions of one variable with appropriate scaling, using equations in x and y and the Cartesian plane
- interpret mathematical relationships both algebraically and graphically
- reduce a given linear equation in two variables to the standard form y=mx+c; calculate and interpret gradients and intercepts of graphs of such linear equations numerically, graphically and algebraically
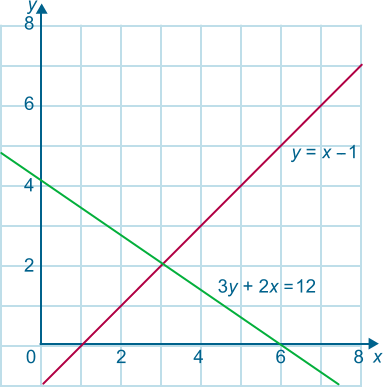
- use linear and quadratic graphs to estimate values of y for given values of x and vice versa and to find approximate solutions of simultaneous linear equations
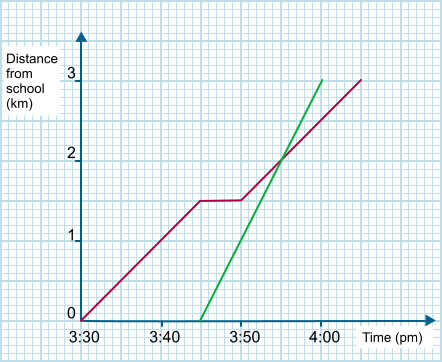
- find approximate solutions to contextual problems from given graphs of a variety of functions, including piece-wise linear, exponential and reciprocal graphs
- generate terms of a sequence from either a term-to-term or position-to-term rule
- recognise arithmetic sequences and find the nth term
- recognise geometric sequences and appreciate other sequences that arise.
 Draw a pentagon and draw in all the diagonals (lines joining the vertices not including the edges of the pentagon), how many diagonals is this?
Draw a pentagon and draw in all the diagonals (lines joining the vertices not including the edges of the pentagon), how many diagonals is this?